Equilateral Triangle Calculator
Equilateral Triangle: It is a type of triangle having three sides of equal length. The interior angles of the equilateral triangle are 60°. The perpendicular line drawn from the vertex of the triangle to the base divides it into two equal parts. The length of that line is called triangle altitude.
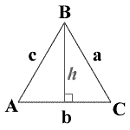
| A = B = C = 60° | |
| a = b = c | |
| side length | a = |
| area | K = |
| perimeter | P = |
| semiperimeter | s = |
| altitude | h = |
Formulas of an Equilateral Triangle
An equilateral triangle is a regular polygon having three sides and three angles equal to 60 degrees. For every equilateral triangle, you can calculate four unknown measurements using one known parameter. The things you can calculate are equilateral triangle area, perimeter, side length, semiperimeter, and altitude. If you know anyone value, then use the below-mentioned formulas to get the other unknown measurements of the triangle.
- The perimeter of an Equilateral Triangle Formula:
- Perimeter P = 3a
- Semiperimeter of an Equilateral Triangle Formula:
- Semiperimeter s = 3a / 2
- The altitude of an Equilateral Triangle Formula:
- Altitude h = (√3 / 2) * a
- Area of an Equilateral Triangle Formula:
- Area K = (√3 / 4) * a²
Equilateral Triangle Calculations:
- If the perimeter of the triangle is known, then
- Side length a = P / 3
- Semiperimeter s = P / 2
- Area K = (3√3 / 4) * P²
- Altitude h = (1 / 2√3) P
- If the semi perimeter of the triangle is known, then
- Side length a = 2s / 3
- Perimeter P = 3a
- Altitude h = (1/2) * √3 * a
- Area K = (1/4) * √3 * a²
- If the area of the triangle is known, then
- Side length a = √( (4 / √3) * K) = 2 * √(K / √3)
- Perimeter P = 6 * √(K / √3)
- Semiperimeter s = 3 * √(K / √3)
- Altitude h = √3 * √(K / √3) = √(3K / √3)
- If the altitude of a triangle is given, then
- Side length a = (2 / √3) * h
- Perimeter P = 3a
- Semicircle s = 3a / 2
- Area K = (√3 / 4) * a²
- If the side length of the triangle is known, then
- Perimeter P = 3a
- Semiperimeter s = 3a / 2
- Area K = (√3 / 4) * a²
- Altitude h = (√3 * a) / 2
Steps to Compute Equilateral Triangle Area, Height, Perimeter
Go through the simple and easy steps of how to calculate the equilateral triangle perimeter, side length, area, semiperimeter, and height. Follow these instructions and find the solution you want.
Equilateral Triangle Perimeter:
- Get the length of the side of a triangle.
- Multiply the side length with 3 to get the perimeter.
Equilateral Triangle Semiperimeter:
- Make a note of the triangle side length.
- Multiply the side length with 3 and divide the result by 2.
- The obtained value is the semiperimeter.
The altitude of an Equilateral Triangle:
- Check the side length of an equilateral triangle from the question.
- Get half of the side length and multiply it by √3.
- The result is the triangle height.
Equilateral Triangle Area:
- Note down the triangle side length.
- Square the side length and multiply it with √3.
- Divide the result by 4 to get the triangle area.
Equilateral Triangle Side Length:
- Process 1:
- Check out the perimeter of the triangle.
- Divide it by 3 to get side length.
- Process 2:
- Get a semiperimeter of an equilateral triangle from the question.
- Double the semiperimeter and divide it by 3 to find a triangle semiperimeter.
- Process 3:
- Note down the height of the triangle.
- Double the height and divide it by √3.
- Process 4:
- Check the area of the equilateral triangle.
- Divide the area by √3.
- Apply the square root function to it.
- Double the result to find the equilateral triangle side length.
Equilateral Triangle Interior Angle
All the angles of an equilateral triangle are equal. The sum of angles of a triangle is 180°. So, each angle gets 60°.
Example Questions on Equilateral Triangle
Example 1: If the equilateral triangle perimeter is 76 m, find its area, semiperimeter, altitude, and side length?
Solution:
Given that,
Equilateral Triangle Perimeter P = 76 m
Side length of an Equilateral Triangle a = P / 3
= 76 / 3
= 25.33
Altitude of a Triangle is h = (1/2) * √3 * a
= (1/2) * √3 * 25.33
= 12.6 * √3
= 21.93
Semiperimeter is s = 3a / 2
= (3 * 25.33) / 2
= 75.99 / 2
= 37.99
Equilateral Trinagle Area is K = (1/4) * √3 * a²
= (1/4) * √3 * 25.33²
= (1/4) * √3 * 641.6
= √3 * 160.402
= 277.82
∴ Equilateral Triangle side length is 25.33 m, area is 277.82 m², perimeter is 76 m, semiperimeter is 37.99 m, height is 21.93 m.
Example 2: Calculate equilateral triangle semiperimeter, perimeter, side length, and height? If the triangle's area is 150 cm².
Solution:
Given that,
Equilateral triangle area K = 150 cm²
Side length a = 2 * √ [ K / √3 ]
= 2 * √ [ 150 / √3 ]
= 2 * √86.60
= 2 * 9.306
= 18.612
Perimeter P = 6 * √(K / √3)
= 6 * √(150 / √3)
= 6 * √86.60
= 6 * 9.306
= 55.836
Semiperimeter s = 3 * √(K / √3)
= 3 * √(150 / √3)
= 3 * √86.60
= 3 * 9.306
= 27.91
Altitude h = √(3K / √3)
= √((3 * 150) / √3)
= √(450 / √3)
= √259.807
= 16.118
∴ The equilateral triangle altitude is 16.118 cm, semiperimeter is 27.91 cm, the perimeter is 55.836 cm, side length is 18.612 cm.
Areavolumecalculator.com has a huge collection of online calculators for plenty of concepts in geometery. Explore it and make your calculations quite easy.
FAQs on Equilateral Triangle Calculator
1. What is Meant by Equilateral Triangle?
An equilateral triangle is a two-dimensional figure having three sides of equal length. The interior angles of an equilateral triangle are also measured equal which is 60°. The sum of the interior angles of a triangle is 180°.
2. How do you find the missing side of an equilateral triangle?
You can calculate the length of the side of an equilateral triangle by using the following formulas.
Side length a = Perimeter / 3
Side length = (2 * semiperimeter) / 3
Side length = 2 * √(Area / √3)
Side length = 2 / √3 (height)
3. How do you find the equilateral triangle area?
Equilateral triangle area formula using pythagorean theorem is area = (side length * height) / 2
Height is h = a * √3 / 2
area = a² * √3 / 4
using trigonometry is area = (1/2) * a * b * sin(γ)
= (1/2) * a * a * sin(60°)
= (1/2) * a * a * (√3 / 2)
= √3 / 4 * (a²)
4. What are the properties of an equilateral triangle?
The basic properties of an equilateral triangle are:
All the sides and angles are equal.
Each interior angle is equal to 60 degrees.
It is a regular polygon with three sides.
